- Home
- Better Memory
- Free Stuff
- Checkerboards
Checkerboards - A Jigsaw Puzzle with Math Facts
Checkerboards puzzle
Let's hear it for Checkerboards, another original math puzzle game created by my friend Whit McMahan. This new game is a printable math puzzle in the genre of Sudoku, Mathdoku, Kakuro, and KenKen.
Whit describes Checkerboards as "jigsaw puzzles, but played with positive and negative numbers." Below there are free Checkerboard puzzle PDFs below for you to print out and try, compliments of Whit himself.
Checkerboards are a twist on Whit's math game Balance Quest. He actually sells a Balance Quest puzzle book on Amazon.com, in case you're interested.
Whit is something of a math whiz. He enjoys exploring properties of mathematics with the goal of making something fun and new. Checkerboards is one of his latest creations.
Free Printable Checkerboards Puzzles
Without further ado, here are the free printable Checkerboards puzzles, arranged by size and difficulty. Instructions on how to solve them follow.
How to Play Checkerboards
The rules of Checkerboards are not complicated. But this game can be fiendishly challenging, especially with the larger size boards. If you've ever tried to solve a sudoku board populated with woefully few entries, you know what I mean. But that challenge is the fun and the skill of it.
Here's how Checkerboards works:
Using simple addition, fill in every shaded box, based on the sum of the numbers in the other shaded boxes.
The darkest shaded boxes, in the corners of the subsections, contain a number between negative eight and positive eight (-8 to 8) for small Checkerboard puzzles.
In the larger size of the puzzle, the dark corner pieces contain a number between negative sixteen and positive sixteen (-16 to 16).
The one exception is the number zero (0). The only box in which a zero can appear is the box in the very center of the puzzle. In fact, a zero must appear there, to prove that the values you entered actually balance the puzzle.
To prove that you've balanced the Checkerboards puzzle, add up the values in the four center gray squares located around the perimeter. They must sum to zero for the puzzle to be balanced and solved.
Checkerboards Example
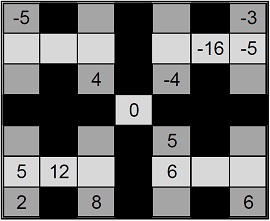
Small Checkerboard Puzzle - Solved
As an example of a correctly balanced puzzle, consider the solved Checkerboards puzzle in the screen image at left. This is the small size puzzle.
The center grays around the perimeter (clockwise from upper left) have been filled in with -2, 4, -8, and 6. If you add these up, you get -2 + 4 = 2 + (-8) = (-6) + 6 = 0. Balanced!
Having jumped right to the solution with that one, I'll next explain how the values you see in the solution were found. Calculating the values of the missing squares and balancing the puzzle is your goal.
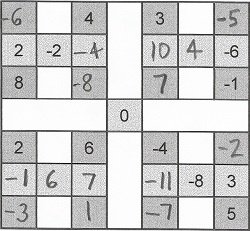
Let's take a look at the upper left subsection. Below a screen image showing the beginning state of this section. Some values are given, the rest you need to calculate.
Notice first the row in the center. Two values are provided (the 2 and the -2), and the third cell starts out blank. To determine the value of the blank cell, ask yourself what number, when added to 2 (the first value), results in a sum of -2 (the middle square in the row)?
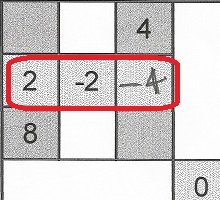
Upper Left: 2 + (-4) = -2
In other words, 2 + _?_ = -2. The only number that satisfies this statement is -4. So I entered -4 in that third square.
Now look at the first column in that subsection. The first cell is blank, the middle cell contains a 2, and the third cell has an 8.
Think to yourself, _?_ + 8 = -6. The only number that satisfies that math statement is -6, and that's what I entered in that cell.
Here's a printable version of the instructions: Checkerboards Puzzle Rules.
To complete the entire Checkerboard puzzle, you proceed in the same manner through the four sections of the puzzle.
Once you've filled out the entire grid, check your work by adding up the values from the center gray squares around the perimeter as I described above. If the center grays add up to zero, you've done it!
More Cool Math Puzzles by Whit
For more puzzles like these as created by Whit McMahan, or to contact Whit directly, be sure to visit his puzzle page and Facebook page. And don't forget to try his original Balance Quest puzzles right here on this site.
Solving math puzzles is not only fun, it's a great way to keep your brain sharp!
Published: 12/29/2014
Last Updated: 06/11/2020

Newest / Popular
Multiplayer
Board Games
Card & Tile
Concentration
Math / Memory
Puzzles A-M
Puzzles N-Z
Time Mgmt
Word Games
- Retro Flash -
Also:
Bubble Pop
• Solitaire
• Tetris
Checkers
• Mahjong Tiles
•Typing
No sign-up or log-in needed. Just go to a game page and start playing! ![]()
Free Printable Puzzles:
Sudoku • Crosswords • Word Search